Materials - Communicate Science

It’s still the holiday season, so no apologies for doodling on about gingerbread, which, as it turns out, can be pretty strong stuff – if a bit bendy.

Cue my wife Erin’s first attempt at a gingerbread house (above). Pretty good, huh? The heat from the incandescent fairy lights has kept it from turning mushy, and nicely spiced up the room at the same time. The house is only eight inches tall, but prompts the obvious question: “How high can you build with gingerbread?”

A structural analysis of a full-on house with walls, windows and doors is too tall an order, even with finite element techniques, so I settled on calculating a ballpark maximum height based on standard engineering equations for a free-standing gingerbread column.

There’s no wind blowing through our lounge, so we can ignore sideways forces and focus on the two likely failure modes a column of gingerbread might suffer – just because of its own weight as it gets taller, i.e.:

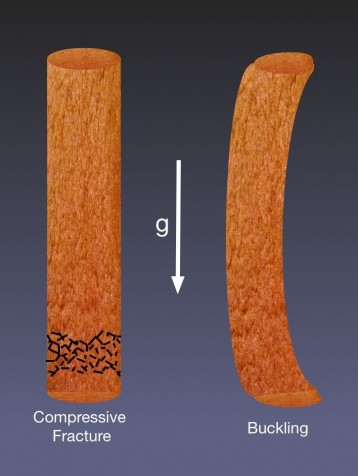(a) the construction materials can disintegrate under their own weight: a function of compressive strength, or

(b) the column can buckle, which is more related to the material’s elastic, or tensile properties.

The heights at which these two failures occur can be found from, respectively:

$H_{max} = \frac {P_f} {\rho\times g}$

$H_c =\left(\frac{9 E I}{4\rho g A}j^2\right)^{(1/3)}$

where $H_{max}$ =column height at compressive failure (m), $P_f$ is the failure pressure (N/m²) = compressive strength of the gingerbread, g=gravity (9.8 ms^-2), and $\rho$ is gingerbread density (kg m^-3). And for buckling: $H_c$ is the critical height, E is Young’s Modulus of elasticity calculated as tensile stress/strain, I is the Area Moment of Inertia³, and $j\approx 1.8663$ is a factor called a Bessel function, used to solve this type of equation (Ref.2)

Using published gingerbread properties data¹ (amazingly, there actually are some) for compressive strength and tensile stress/strain, I calculated values of:

$H_{max} = 50metres$

$H_c=3metres$

(Workings in box below if you’re interested.)

which essentially means a gingerbread column will start to lean over and buckle sideways long before the gingerbread breaks up through compression under its own weight (I used an arbitrary but realistic 20 cm column diameter). You might think there’s no reason why a uniform, vertical, column would start to lean, but in real life the weight distribution is never uniform and, if the column is sufficiently slim, a turning moment will establish and drive a progressive buckle.

So if you’re going to build a gingerbread house out of free-standing columns, better stop at 3 metres.

Buckling is clearly the limiting factor, but the 3 metre figure is based on a relatively small 0.2m column diameter, and buckling is particularly sensitive to cross-sectional area (whereas compressive fracture of a column under its own weight is independent of area). Also, most real buildings are more complex than a bunch of pillars, and I’d expect the right combination of interconnecting members building up from a broad foundation could reduce buckling potential, making a full-size gingerbread house a reality.

Indeed, the Guinness Book of Records ‘Worlds largest gingerbread house’ is 18.28m (60ft) on a 13.86m by 10.8m base; but closer inspection shows it’s built around a steel frame that presumably keeps incipient buckling in check. But then it’s more of a gingerbread and steel house – a bit of a con really.

Anyhow, our room’s about 3 metres high, so nothing stopping a more ambitious project next year: Empire State Building or Cathédrale Notre Dame ?

Workings

Note that for compressive failure of a column under its own weight, the area of the column A (m²) cancels and isn’t relevant: i.e $P_f = \frac {m\times g}A$ so, $P_f * A = H_{max} A\times \rho\times g$ and $H_{max} = \frac {P_f} {\rho\times g}$ as above.

I couldn’t measure my own gingerbread density easily (although it for sure floated in water, so < 1000 kg m^-3), and used a middle value of 700 kg m^-3 from this unlikely study by students at the University of British Columbia (UBC)¹. In addition to the UBC data for $P_f$ of 346 kPa, I measured my own value for $P_f$ by pressing a sample (squirrel-shaped in this case, but taking the narrowest foot area as 1*10^-4m²) vertically downwards onto a balance and recording when it crumbles.Still intact when the balance read 6kg, I took my $P_f$ to be at least 6 * 9.8 / 1*10^-4, or 588 * 10³ N/m² (588 kpascal kPa). In fact, for compressive strength, my numbers and the published data are conservative, as in neither case did the gingerbread actually fail at these values. So:

$H_{max} = \frac {346\times 10^3}{700\times 9.8}=50 m$ (UBC data)

$H_{max} = \frac {588\times 10^3}{700\times 9.8}=85 m$ (my gingerbread)

Whether it buckles first, at a lower height, depends on the elasticity of the gingerbread and the slenderness of the column: i.e. the ratio of column area to length.

The height at which buckling occurs can be found from Cox & McCarthy²:

$H_c =\left(\frac{9 E I}{4\rho g A}j^2\right)^{(1/3)}$

where $H_c$ is the critical height for buckling, E is Young’s Modulus of elasticity calculated as tensile stress/strain, and I is the Area Moment of Inertia³.

To calculate $H_c$ , I chose an arbitrary column diameter of 20 cm diameter, and used stress/strain data from the Canadian study¹ to calculate E = 9790 kPa; i.e. 219/0.02237 (the change in dimensions of my squirrel under tension are too small to measure with the kit I have).

The Area Moment of Inertia for a circular cross-section $I = \frac{\pi}{4} r^4$ , which for a 0.2m dia. column gives $I= 7.85\times 10^-5$ . And ( $j\approx 1.8663$ , is the appropriate Bessel function of order -1/3 (Ref.2) Note: in the source equation, weight density is specified; hence g added here.) So:

$H_c =\left(\frac{9\times 9790\times 10^3\times7.85\times 10^-5}{4\times 700 \times 9.8\times \pi\times 0.1^2}\times1.8663^2\right)^{(1/3)}$

$H_c = 3 metres$

A more complex structure would best be assessed computationally using finite element analysis, but I’m not getting into that.

References
1. ‘Building with gingerbread: Engineering students put holiday delight to the test’ refers to ‘Structural Analysis of Gingerbread. Engineering Design Project Term 2’ by Mercedes Duifhuis and Sean Heisler (pdf)
2. The Shape of the Tallest Column. Steven J.Cox, C.Maeve McCarthy, Society for Industrial and Applied Mathematics. Vol29,No.3. pp.547-554. (Also see Wikipedia page on buckling.)
3. Engineering Fundamentals efunda.com/math)

Of related interest
How to create the perfect sand castle Nature Scientific Reports 2, Article number:549, doi:10.1038/srep00549

Communicate Science

Category Archives: Materials

Musings on Structural Gingerbread

Zoonomian Blog